Phase Retrieval for Fourier Ptychography under varying amount of measurement
In BMVC 2018 [paper] [code]
Lokesh Boominathan, Mayug Manipirambil, Honey Gupta, Rahul Baburajan, Kaushik Mitra
Abstract
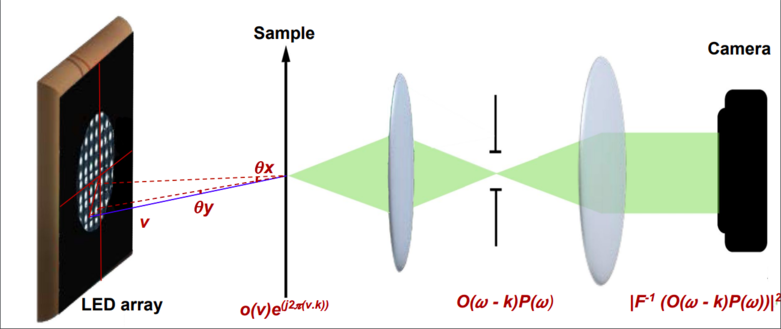
Fourier Ptychography Forward Model
- LED illumination: Let us consider the imaging of a thin target sample having transmission function given by $o(v)=A(v)\mathrm{e}^{j\phi(v)}$, where $v$ is the spatial vector, and $A(v)$ and $\phi(v)$ are the amplitude and phase attenuation respectively. If the target sample is illuminated using an LED that emits light with spatial frequency $k$, then light from the target sample that reaches the objective lens is $o(v)\mathrm{e}^{j2\pi(v.k)}$. Therefore, if $O(\omega)$ is Fourier transform of the target sample under normal illumination, then the Fourier transform under angular illumination would be $O(\omega-k)$.
- Objective lens: Let $P(\omega)$ be the pupil function of the objective lens, then the light passing through it would be $O(\omega-k)P(\omega)$. Since under normal illumination $P(\omega)$ acts as a low pass filter with cut-off frequency given by its numerical aperture, under angular illumination it would behave as a band pass filter.
- Image acquisition: However, since camera sensors can capture only the intensity and not the phase of light coming from the objective lens, the captured image is given as $|{\mathcal{F}}^{-1}(O(\omega-k)P(\omega))|^2$, where ${\mathcal{F}}^{-1}$ is Fourier inverse. Multiple such images are taken using varying angles of illumination to capture different regions of the object's Fouier domain.
Our Contribution
Method
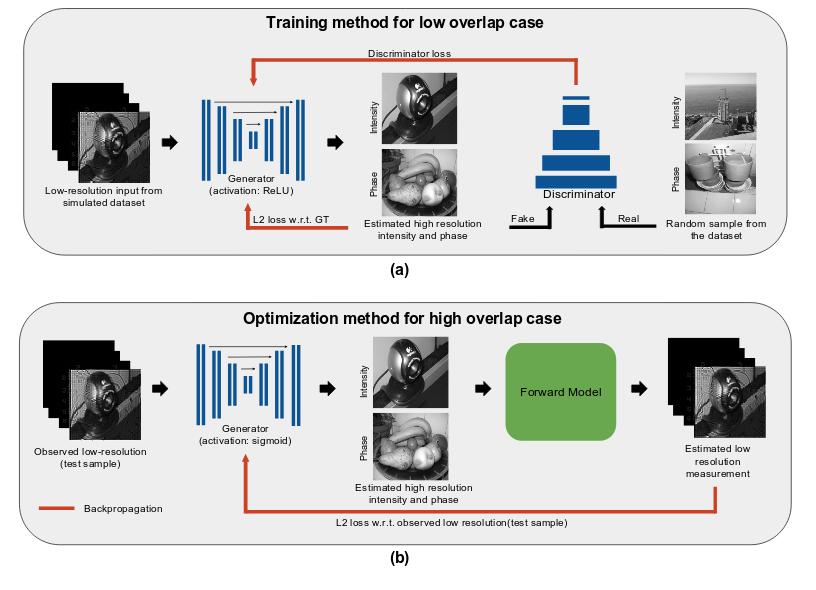
Results
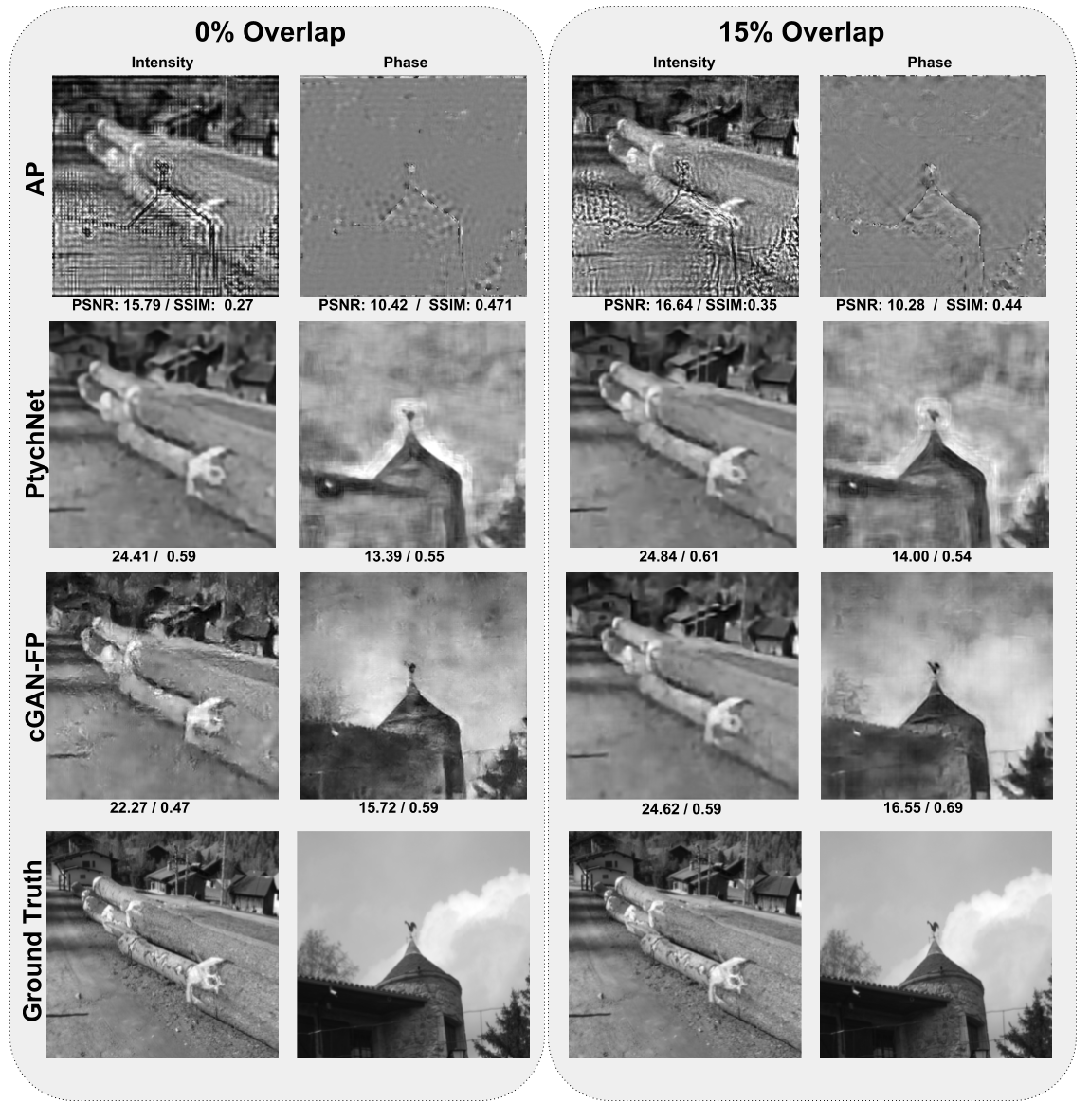
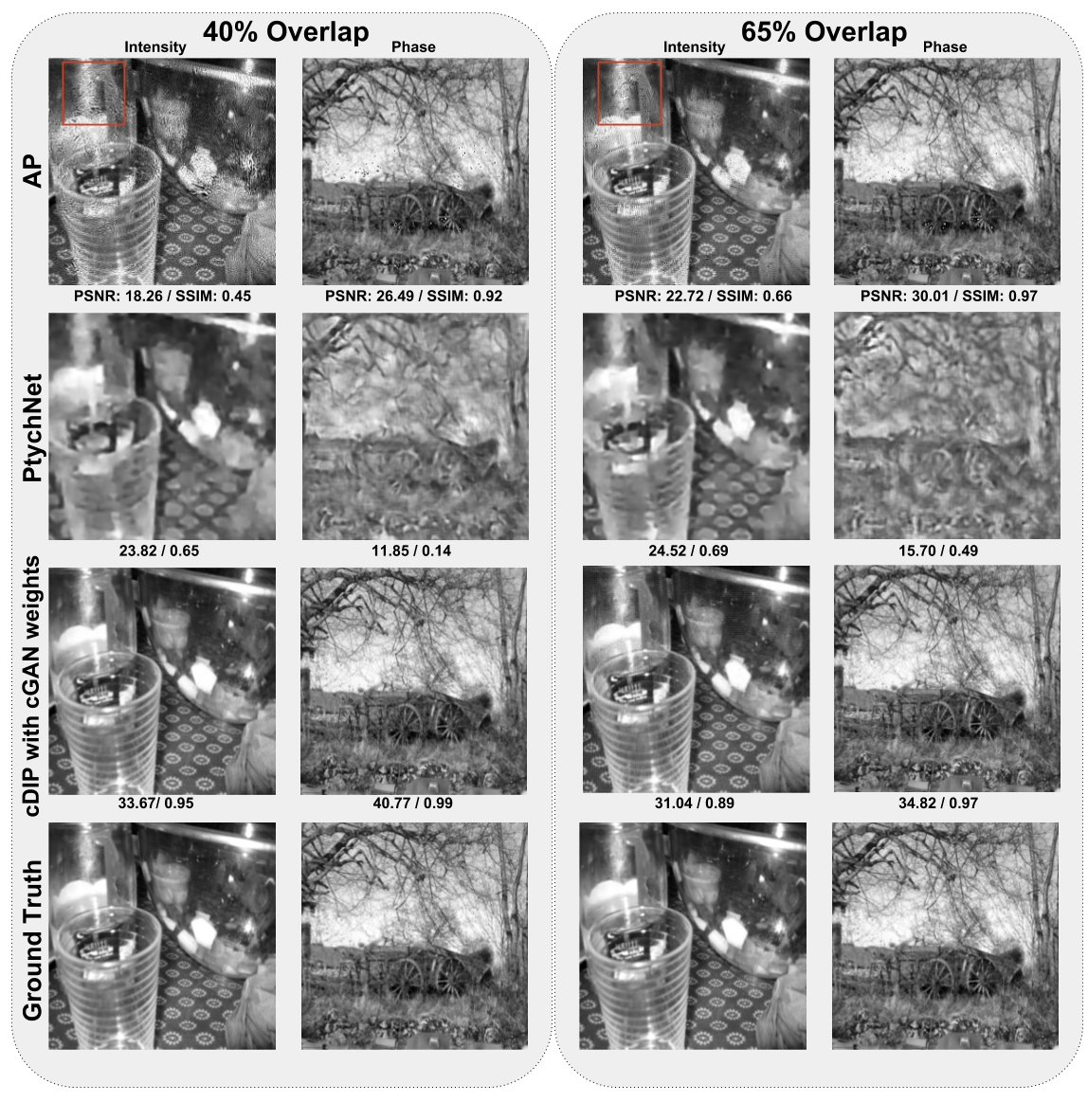
Methods in the comparison
- RW Gerchberg. Super-resolution through error energy reduction. Optica Acta: International Journal of Optics, 21(9):709–720, 1974.
- James R Fienup. Reconstruction of an object from the modulus of its fourier transform. Optics letters, 3(1):27–29, 1978.
- Armin Kappeler, Sushobhan Ghosh, Jason Holloway, Oliver Cossairt, and Aggelos Katsaggelos. Ptychnet: Cnn based fourier ptychography. In Image Processing (ICIP), 2017 IEEE International Conference on, pages 1712–1716. IEEE, 2017.